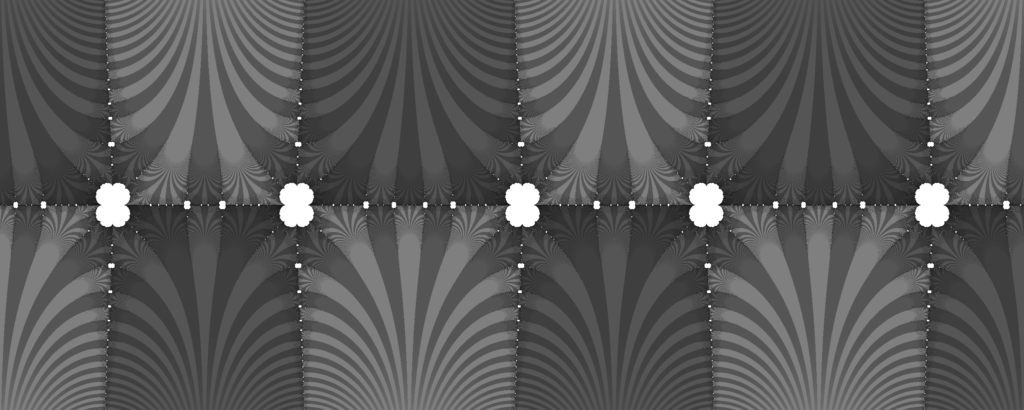
wandering domains for the entire function f(z)=z+2πsin(z) (source Wikipedia from no-wandering-domain theorem)
04_2017
+++++++++++++++++++++++++++++++++++++++++++++++++++
The concept of a wandering set formalizes a certain idea of movement and mixing in such systems. When a dynamical system has a wandering set of non-zero measures, then the system is dissipative. This is very much the opposite of a conservative system, for which the ideas of the Poincaré recurrence theorem apply. Intuitively, the connection between wandering sets and dissipation is easily understood: if a portion of the phase space “wanders away” during normal time-evolution of the system, and is never visited again, then the system is dissipative. The language of wandering sets can be used to give a precise, mathematical definition to the concept of a dissipative system. The notion of wandering sets in phase space was introduced by George David Birkhoff in 1927.
extracts: